About Chandra Education Field Guide Photo Album Press Room Resources Multimedia Podcast

Keep Up With Your Math, and Give Physics A Try Too

December 11, 2002 ::

Chandra Supernova Remnants UL: G292.0+1.8 UR: Cassiopeia A LL: 3C58 LR: N132D

As part of a class project, a student from Miami, FL, recently sent the Chandra X-ray Center a series of questions on how trigonometry is used in astrophysics. Trigonometry is the branch of mathematics that deals with the relationships between the sides and angles of triangles, and the calculations based on them. The following text includes replies from Dr. Patrick Slane, a scientist who uses and works for the Chandra X-ray Observatory.

Q: My name is Fernando and I live in Miami, Fl. I would like to ask an Astrophysicist some general questions about how Trigonometry is used in his/her profession. This is part of a project I am doing for my Trigonometry class about professions that use Trigonometry.

A: My name is Pat, and I'm an astrophysicist at the Harvard-Smithsonian Center for Astrophysics in Cambridge, MA. I study supernova remnants and neutron stars, both of which are relics of massive star explosions. I also work on the Chandra X-ray Observatory, helping to plan observations and operate the spacecraft. I live outside of Boston, MA, where it is presently quite a bit colder than in Miami, and where the Patriots currently share the AFC East lead with your Dolphins.

Illustration of Chandra's Orbit

Q: Would trigonometry be an essential branch of math for someone wanting to be an astrophysicist?

A: Absolutely.

Q: Do you use trigonometry very much in your profession?

A: Yes, and the range of problems for which it is important is vast. Trigonometry plays a role any time orientation is important. Here are a couple of examples from my work: using trigonometry to calculate distances to objects in the Galaxy, figuring out the effects of magnetic fields on charged particles. (For example, electrons feel a force when they move perpendicular to a magnetic field, but not parallel to it. Therefore, it is important to calculate how much of the velocity is perpendicular to the field.) And, scientists also use trigonometry to calculate the orbit of a moving spacecraft.

Q: Is trigonometry the kind of math you use most as an astrophysicist or is there another?

A: Since math builds so much on previous math, that's a bit hard to say. For example, you are now studying trigonometry, but I'll bet you are using more algebra than trigonometry in your work. That is because we use algebra as tool to make use of the trig. In fact, you probably use arithmetic even more. It's the same with the work I do. I use calculus and some other advanced mathematics techniques to do some calculations. (This will vary from researcher to researcher; someone who works on theoretical astrophysics will tend to use more calculus, complex variable analysis, and maybe numerical analysis, than someone who does more observational and interpretive work, which is what I do.) I use algebra, trigonometry, and analytic geometry to do lots of calculations and modeling of data. In the end, I use a ton of arithmetic to put the numbers together and come up with answers.

Crab Nebula with Scale

Q: Can you give an example of a problem in astrophysics that can be solved using trigonometry?

A: I'll give you a simple one. Let's look at a Chandra image of the Crab Nebula. This is a bubble of energetic electrons driven as a wind from a rapidly rotating pulsar (it rotates 30 times a second, has a mass about 1.5 times that of the Sun, and a magnetic field about 10¹² - that's ten to the twelfth power, or 1,000 billion - times stronger than that of the Earth). We believe that the wind forms a circular ring of emission where it starts to merge with the flow in the rest of the Nebula. You can see the inner ring in this image, as well as the compact pulsar, as a dot in the center. The ring certainly doesn't look circular, but we think that's because it is tilted away from us. You can use Trigonometry to calculate the tilt angle:

• Measure the radius of the long axis of the inner ring, A, and also the short axis, B. (It doesn't matter what units you use because you are going to take the ratio of these anyway, and the units will cancel. So measure it in centimeters on the image, for example.)
• The tilt angle, theta, (or inclination angle, as we call it) is related to these by cos(theta) = B/A. That is, if theta = 0 (so that the ring is not tilted at all), we expect B = A, and as theta approaches 90 degrees, the projected size of the short axis approaches zero. The image indicates that the ring is tilted so that the lower left is farther from us than the upper right.

Crab Nebula Optical Image

Too simple? Ok, then consider this: We know from detailed radio and optical observations that the Crab Pulsar is actually moving across the sky. For example, pictures taken with the Hubble Space Telescope show that the pulsar moved about 34.2 milliarcseconds (that's 9.5 millionths of a degree - quite a telescope that Hubble is!) over the course of 1.9 years. You can use Trigonometry to turn that into an actual speed if you know the distance, D, to the Nebula. We believe this distance is 2 kiloparsecs (more geometry and trigonometry goes into that definition of a distance - look it up for extra credit!) or 6.2 x 10²¹ cm in more normal units.

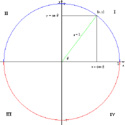• If the angle that the pulsar moves across the sky is phi = 9.5 x 10^-6 degrees in that time, that's a distance of d = D*tan(phi). That gives a velocity of v_x = d/t where t = 1.9 years (convert it to seconds). I get about 170 km/s (yes, I converted it to kilometers - just to make you work harder!). Note: I called this v_x because it is only one component of the velocity. Keep reading...

Now look back at the image of the Crab Nebula. You can see a jet of material "spraying" out of the pulsar, directed to the southeast (that's the lower left in the figure) and the basic form of a counter-jet that extends in the opposite direction. The interesting thing is that the direction of the pulsar motion is along that counter-jet direction (that is, the pulsar is moving toward the upper right in the figure).

What does this have to do with the tilt angle you just calculated? Well, we assume that the pulsar jet is actually perpendicular to the inner ring, which means it is tilted into the plane of the sky by the same tilt angle. The velocity you just calculated is only the component along the plane of the sky.

That's not the complete velocity because it doesn't include any motion directly along our line of sight. But you can now calculate it! You have the x component of the velocity and the angle, so you can get the total velocity since v_x/v = cos(theta) or v = v_x/cos(theta).

Knowing how fast the pulsar is moving tells us something about the kick it received when it was formed in a supernova explosion. Knowing that it is moving along the direction of the jet is presumably telling us something about the formation of the jet as well. People are still working on what it all means.

Last exercise: We know the Crab was formed in an explosion in July 1054 A.D., because Chinese astronomers recorded it. How far has it moved from its birthplace in that time?

By the way, have a look at: http://chandra.harvard.edu/photo/0052/index.html for lots more information and images about the Crab.

Q: Do you enjoy using Trigonometry?

A: I do. It was one of my favorite classes in High School and I still enjoy it.

Q: Out of all six Trigonometric functions, which one is your personal favorite?

A: I guess that's got to be the tangent because that relates to other things in spherical geometry (and literature: "This astrophysicist really seems to have gone off on a tangent about pulsars.").

Q: Which is your least favorite?

A: Oh, I don't discriminate amongst the rest. I don't really ever use the secant or cosecant because they are just inverses of the sine and cosine functions.

Q: When did you last use Trigonometry (before this interview)?

A: Last Saturday. (That's something else you need to know about astrophysicists. When we are supposed to be helping to clean the house on a Saturday morning, sometimes we are squirreled away working on some calculations instead...)

Keep up with your math, and give physics a try too. Fun stuff.

Subscribe